Bandspread Calculations

Part 2

We ended part one stating that we could now calculate padders and trimmers, and from there determine what value to use for an inductor. Real tank circuits will always have some stray capacitance, and therefore we need to take it into account.

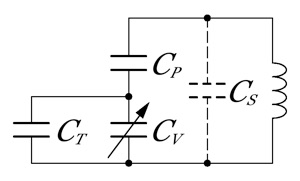Hence, our tank circuit will now include C_S as shown in this diagram. In Part 1, we used the notation C_H and C_L to denote the maximum and minimum values of the tuning capacitor C_V. We also used “prime” notation to denote the net maximum and minimum values after including the trimmer and padder, which we will reiterate here:

C_H , C_L – The maximum and minimum capacitances of the variable capacitor C_V

C_H′ , C_L′ – The maximum and minimum net capacitances when including the (parallel) trimmer

C_H″ , C_L″ – The maximum and minimum net capacitances when including both the (parallel) trimmer and (series) padder.

And, we will now add a third:

C_H‴ , C_L‴ – The maximum and minimum net capacitance when including the trimmer, padder and stray capacitance. Hence, it is these new terms that will be used in the resonance formula to determine inductance and frequency.

Repeating the previous definitions for the above:

Hence:

(6)

And likewise:

Hence:

(7)

By including stray capacitance, the calculations to determine trimmer and padder values become somewhat more complex. We will work through them again, and in addition, we will include the situation where we select the tank inductance L, and then determine the required trimmer and padder values.

Starting with equation (6) and rearranging it:

Let:

Then substituting:

and rearranging

(6a)

Similarly, we can rearrange equation (7) to get:

(7a)

where:

Now subtract (7a) from (6a) to get:

(8)

This allows us to get rid of the C_TC_P term which would otherwise make things a bit messy. And, in order to keep things from getting too unwieldy, we will define the following constants:

and substitute them into (8) to get:

(8a)

Or, expressing the padder in terms of the trimmer:

(8b)

And consequently:

(8c)

We now have a simple expression relating C_P to C_T.

We can use expressions (8b) and (8c) to solve for one when the other is known as we did in Part 1 of this discussion. Having determined both C_P and C_T, we can then substitute these back into the previous formulae to solve for inductance.

Now, what if we want to select an arbitrary value of inductance, and then determine the correct trimmer and padder capacitors to give the desired bandspreading? To do this, we will start with (6a) again:

(6a)

We will define two more constants:

Substituting these into (6a):

Substituting the expression for C_P in terms of C_T from (8b):

Multiplying out the terms:

Then grouping by terms of C_T:

(9)

Once again we have a quadratic equation in the form

where

the coefficients are:

And the solution for C_T is:

(10)

Therefore, we can now solve equation (10) to find C_T, and then substitute the calculated value of C_T back into (8b) to find C_P.

Example

Again we will pick a frequency range of 5500 to 7000 kHz, and tuning capacitor range: C_L =50, C_H =500. We will also assume a stray capacitance C_S=15 pF. For an inductance we will choose L=12 µH.

Referring to resonance formula (1), and remembering that the lowest frequency corresponds to the maximum net capacitance, we get:

Rearranging:

For F_L=5500, we get:

Similarly, for the maximum frequency F_H=7000, we get:

Calculating the constants:

From these we calculate the quadratic coefficients:

Then C_T can be calculated:

And this value of C_T can be substituted into (8b) to find C_P:

These values can be checked by substituting them back into formulae (6) and (7) to get the net circuit capacitances and then into the resonance formula (1) to verify the frequency range. That will be left as an exercise for the reader. The following dial scale shows the tuning characteristic when using a standard midline variable capacitor.

Summary

Given a desired frequency range to bandspread, a tuning capacitor with a given capacitance range, and accounting for stray circuit capacitance, we can now pick either a trimmer, a padder or an inductance value. Then, with the given component value, we can calculate the remaining two unknown component values. As mentioned at the end of Part 1 of this discussion, there is an on-line Bandspread Calculator here, which you can use to perform the calculations discussed above. The calculator determines the values of the two unknown components, when given the value of the known component. While I have not revisited the derivations of Part 1 to include stray capacitance for the cases of fixed Trimmer or fixed Padder, they are actually simpler than the fixed inductor case, and I will address them in a future update of this page. However, the calculator does perform all of these calculations while including the effect of stray capacitance.

In Part 3 we will discuss a method of bandspreading where the  variable bandspread capacitor is connected to a tap on the tank inductor, and a bandset capacitor (either fixed or variable) is connected across the entire coil. This is a method which dates back to the early days of radio, and was used in both commercial and homebrew shortwave radio circuits.

Continue to: Part 3